Saturday, August 14, 2010

Raoult's law (Statement, Derivation, negative and positive deviations,Concept of mixing of ideal and non ideal solutions)


Raoult's law

  Raoult's law states:

 The vapor pressure of an ideal solution is dependent on the vapor pressure of each chemical component and the mole fraction of the component present in the solution.

Expressed as,


 

where
pi is the partial pressure of the component i in mixture
p*i is the vapor pressure of the pure component i
xi is the mole fraction of the component i in solution (in mixture)

Derivation:

We define an ideal solution as a solution for which the chemical potential of component i is:
\mu _i = \mu_i^{\star} + RT\ln x_i\,,
where µ*i is the chemical potential of pure i.
If the system is at equilibrium, then the chemical potential of the component i must be the same in the liquid solution and in the vapor above it. That is,
\mu _{i,{\rm liq}} = \mu _{i,{\rm vap}}\,
Assuming the liquid is an ideal solution, and using the formula for the chemical potential of a gas, gives:
\mu _{i,{\rm liq}}^{\star} + RT\ln x_i = \mu_{i,{\rm vap}}^\ominus + RT\ln \frac{{f_i }} {{p^\ominus }}
where ƒi is the fugacity of the vapor of i and ^\ominus indicates reference state.
The corresponding equation for pure i in equilibrium with its (pure) vapor is:
\mu _{i,{\rm liq}}^{\star} = \mu _{i,{\rm vap}}^\ominus + RT\ln \frac{{f_i^{\star}}} {{p^\ominus }}
where * indicates the pure component.
Subtracting both equations gives us
RT\ln x_i = RT\ln \frac{{f_i }}{{f_i^{\star} }}
which re-arranges to
f_i = x_i f_i^{\star}
The fugacities can be replaced by simple pressures if the vapor of the solution behaves ideally i.e.
p_i \approx x_i p_i^{\star}
which is Raoult’s Law.

Ideal mixing

An ideal solution can be said to follow Raoult's Law but it must be kept in mind that in the strict sense ideal solutions do not exist. The fact that the vapor is taken to be ideal is the least of our worries. Interactions between gas molecules are typically quite small especially if the vapor pressures are low. The interactions in a liquid however are very strong. For a solution to be ideal we must assume that it does not matter whether a molecule A has another A as neighbor or a B molecule. This is only approximately true if the two species are almost identical chemically. We can see that from considering the Gibbs free energy change of mixing:
\Delta_{\rm mix} G = nRT(x_1\ln x_1 + x_2\ln x_2)\,
This is always negative, so mixing is spontaneous. However the expression is, apart from a factor –T, equal to the entropy of mixing. This leaves no room at all for an enthalpy effect and implies that ΔmixH must be equal to zero and this can only be if the interactions U between the molecules are indifferent.
It can be shown using the Gibbs–Duhem equation that if Raoult's law holds over the entire concentration range x = 0–1 in a binary solution then, for the second component, the same must also hold.
If the deviations from ideality are not too strong, Raoult's law will still be valid in a narrow concentration range when approaching x = 1 for the majority phase (the solvent). The solute will also show a linear limiting law but with a different coefficient. This law is known as Henry's law.
The presence of these limited linear regimes has been experimentally verified in a great number of cases.
In a perfectly ideal system, where ideal liquid and ideal vapor are assumed, a very useful equation emerges if Raoult's law is combined with Dalton's Law.
x_i = \frac{y_i p_{\rm total}}{p_{i,{\rm eqm}}}\,

Non-ideal mixing

Raoult's Law may be adapted to non-ideal solutions by incorporating two factors that will account for the interactions between molecules of different substances. The first factor is a correction for gas non-ideality, or deviations from the ideal-gas law. It is called the fugacity coefficient (φ). The second, the activity coefficient (γ), is a correction for interactions in the liquid phase between the different molecules.
This modified or extended Raoult's law is then written:
p_{i} \phi_i = p_i^{\star} \gamma_i x_i\,

Real solutions

Many pairs of liquids are present in which there is no uniformity of attractive forces i.e. the adhesive & cohesive forces of attraction are not uniform between the two liquids, so that they show deviation from the Raoult's law which is applied only to ideal solutions.

Negative deviation

When adhesive forces between molecules of A & B are greater than the cohesive forces between A & A or B & B, then the vapor pressure of the solution is less than the expected vapor pressure from Raoult's law. This is called as negative deviation from Raoult's law. These cohesive forces are lessened not only by dilution but also attraction between two molecules through formation of hydrogen bonds. This will further reduce the tendency of A and B to escape.

 For example, chloroform and acetone show such an attraction by formation of a hydrogen bond.

 

                                                Positive deviation

 When the cohesive forces between like molecules are greater than the adhesive forces, the dissimilarities of polarity or internal pressure will lead both components to escape solution more easily. Therefore, the vapor pressure will be greater than the expected from the Raoult's law, showing positive deviation. If the deviation is large, then the vapor pressure curve will show a maximum at a particular composition, 

 

e.g. benzene & ethyl alcohol, carbon disulfide & acetone, chloroform & ethanol.


 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

at
Labels:

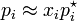
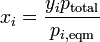
No comments:

Post a Comment

Subscribe to: Post Comments (Atom)

Thermal conductivity calculations, experiments, molecular simulations

Nowadays various experimental procedures are there to calculate the thermal conductivity of various materials using various techniques. Th...

About Me

COTACT: studymaterialforall@gmail.com